Compression characteristics and models of cohesionless soil
-
摘要:
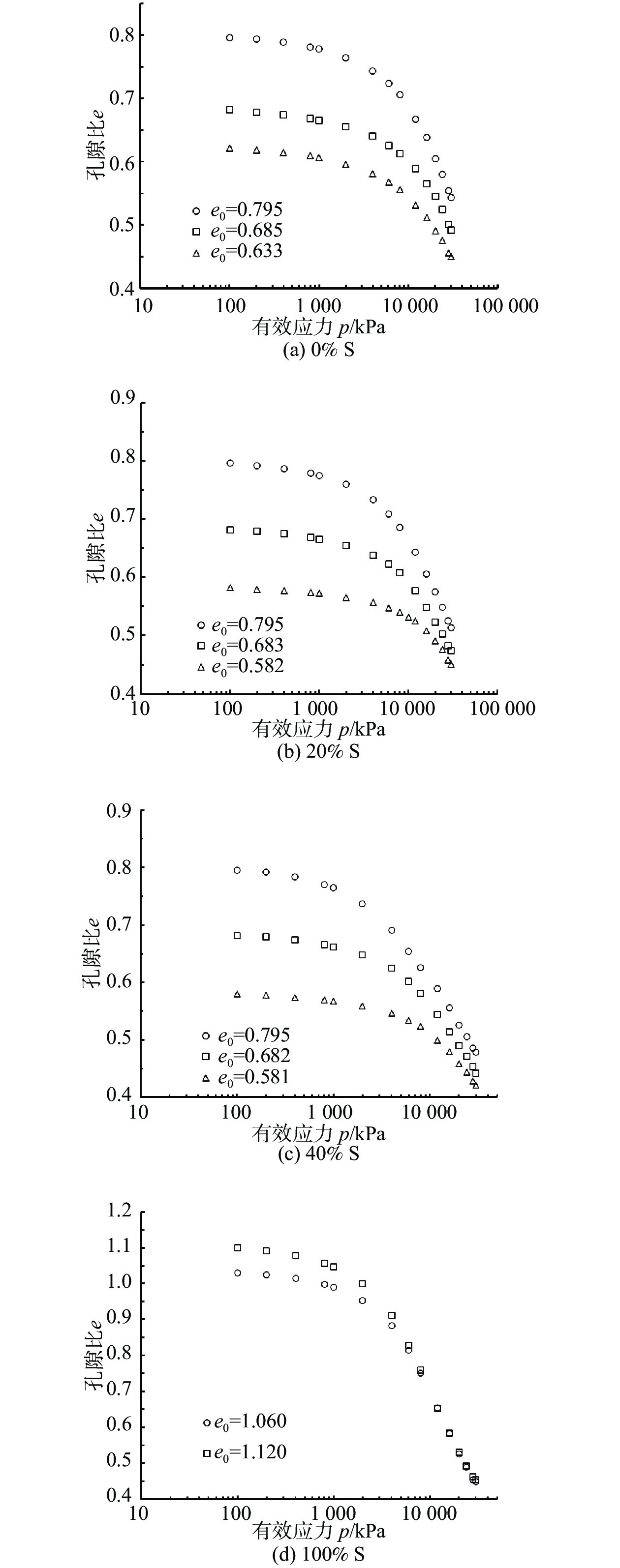
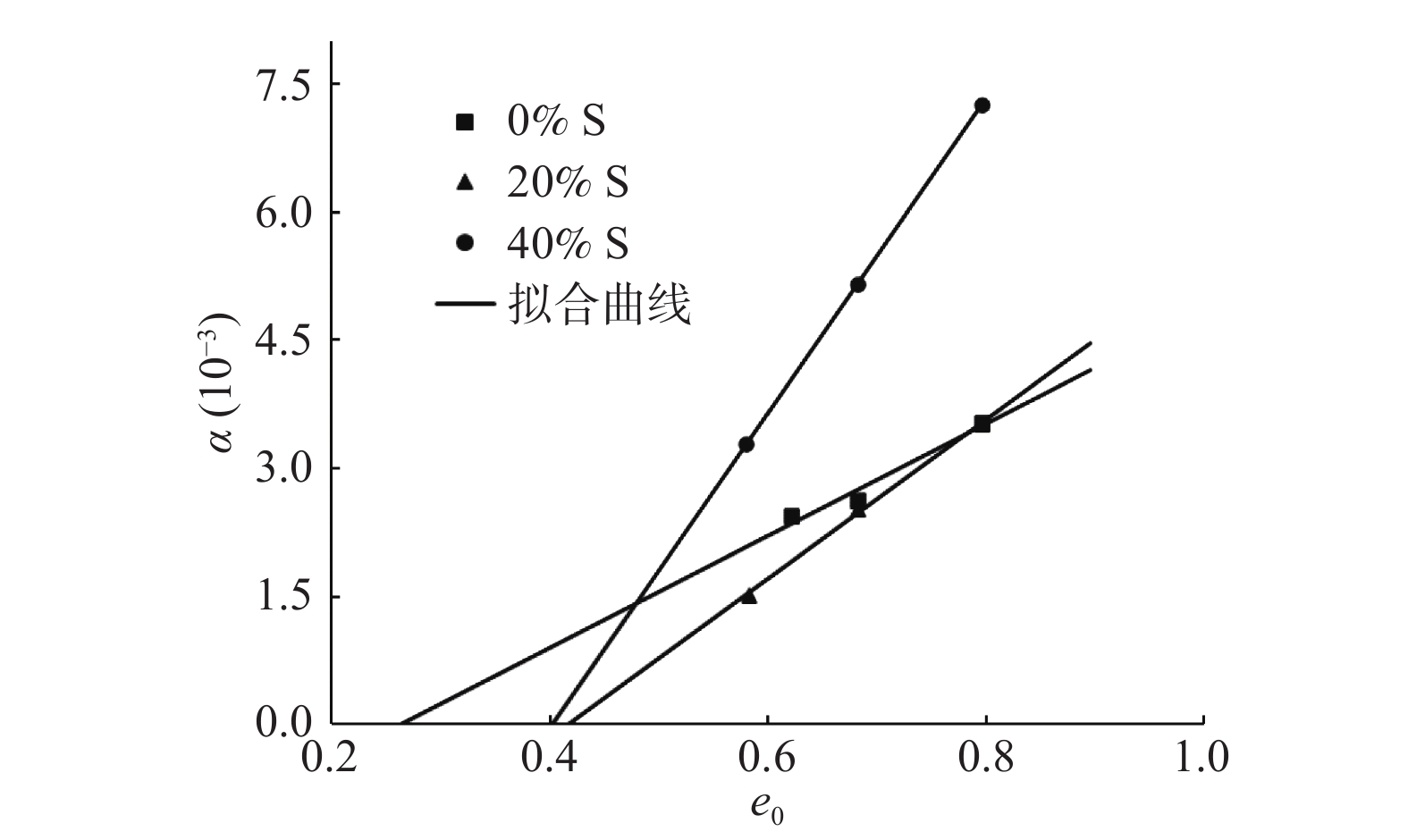
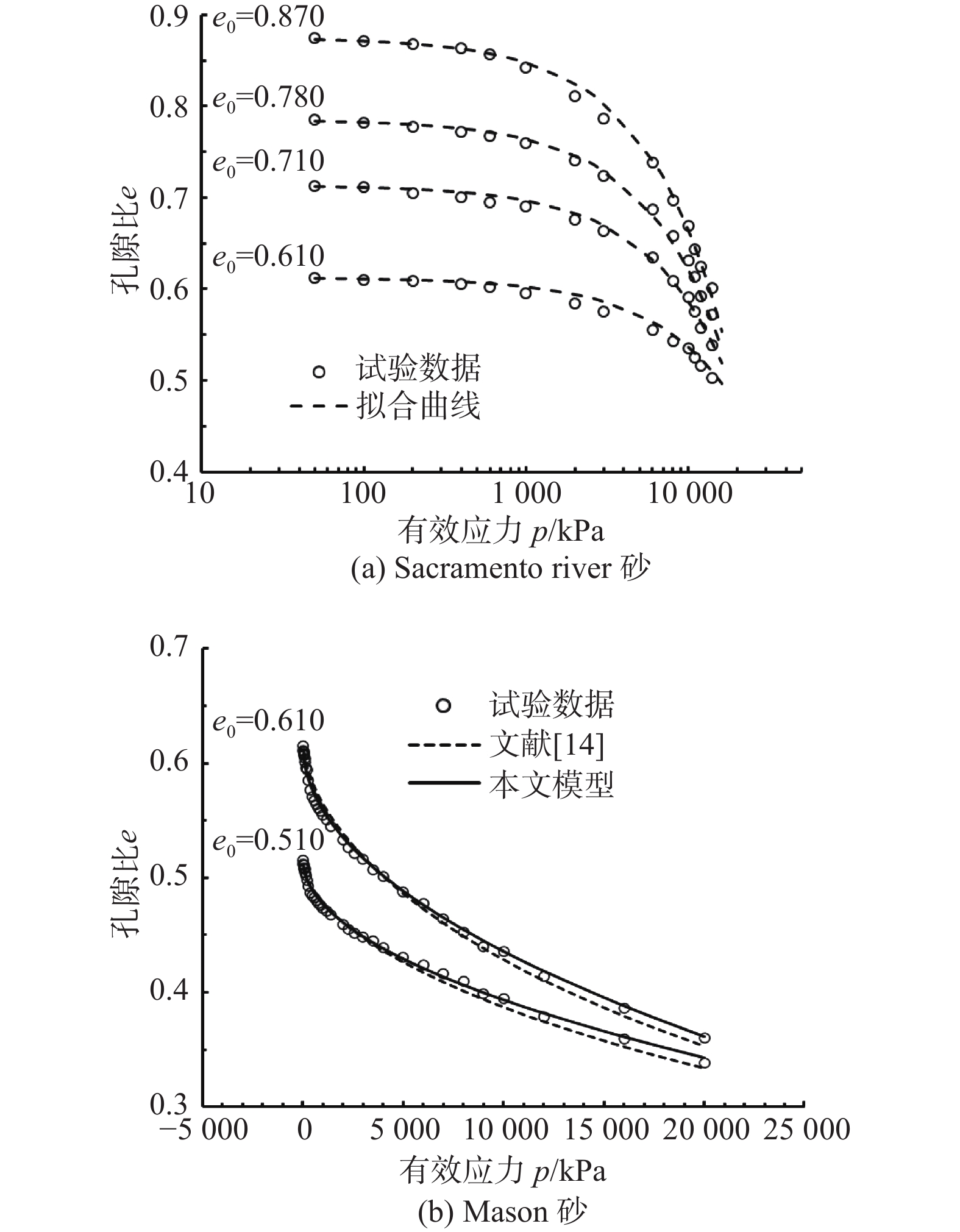
我国南海神狐海域海底沉积物主要由钙质砂与无黏性土组成,其力学性质对海洋工程的稳定性具有显著影响。无黏性土的压缩特性是研究其力学性能的重要内容之一,为分析不同荷载作用下土样的压缩特性,利用高压三轴仪试验系统,开展了不同砂含量及不同初始孔隙比下无黏性土样的等向压缩试验。试验结果表明:在试验采用的高有效应力下,无黏性土具有显著的过渡土性质,初始组构难以被改变;随多孔易碎钙质砂含量的增加,土样可压缩性和压缩曲线的收敛度均增加,钙质砂的破碎显著改变了初始组构。提出可以描述含砂无黏性土压缩特性的数学模型,所含参数物理意义明确且易于确定。与不同砂土压缩试验数据对比发现,该模型对其他种类土同样具有较好的拟合度,验证了本模型的广泛适用性。与已有压缩模型的对比,验证了本模型的实用性,为无黏性土应力-应变关系的理论研究提供基础。
Abstract:The sediments in the Shenhu sea area of the South China Sea is composed of sand and cohesionless soil, and their mechanical properties have a significant impact on the stability of ocean engineering. The compression characteristics of the cohesionless soil with sand is one of the important contents of the studies of its mechanical properties. In order to analyze the compression characteristics of soil samples under different loads, the isotropic compression tests of cohesionless soil samples with different sand contents and different initial void ratios are carried out by using the high-pressure triaxial apparatus test system. The test results show that under the high effective stress, the cohesionless soil has significant transition soil properties, and the initial fabric is difficult to be changed. With the increase of the content of the porous fragile calcareous sand, the compressibility and the convergence of the compression curve of the soil sample increase, and the crushing of calcareous sand significantly changes the initial fabric. A mathematical model is proposed to describe the compression characteristics of the cohesionless soil with sand, and the physical meaning of fitting parameters is clear and easy to determine. Compared with the different soils compression tests data, it is found that the model results also are in a good agreement with those of other kinds of sands, which verifies the extensive applicability of the model. Compared with the existing compression models, the practicability of the model is verified. The results can provide a basis for analyzing the stress-strain relationship of cohesionless soil.
-
Key words:
- carbonate sand /
- cohesionless soil /
- compression model /
- void ratio
-

-
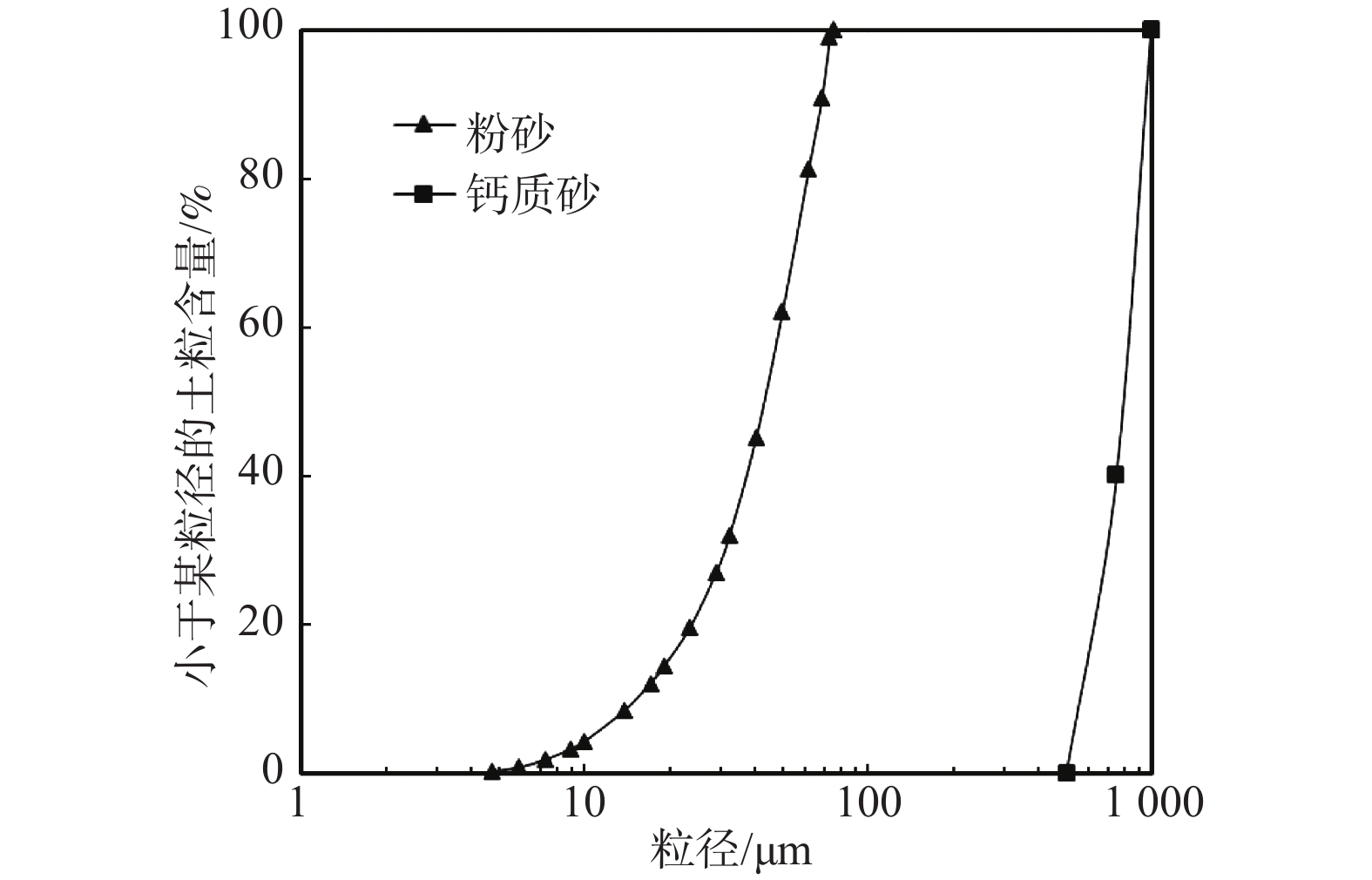
表 1 钙质砂和粉砂的物理参数
Table 1. Parameters of calcareous sand and silt
土类 比重Gs 粒径/mm 最大孔隙比emax 最小孔隙比emin 粉砂 2.63 <0.075 0.880 0.560 钙质砂 2.77 0.5~1 1.290 0.930 表 2 试验方案
Table 2. Test scheme
编号ID 砂含量S/% 初始孔隙比e0 相对密实度Dr/% 加载梯度/MPa 1 0 0.633 90 0.1,0.2,0.4,0.8,
1,2,4,6,8,12,
16,20,24,28,302 0 0.685 80 3 0 0.795 60 4 20 0.582 80 5 20 0.683 60 6 20 0.795 40 7 40 0.581 60 8 40 0.682 4% 9 40 0.795 20 10 100 1.060 80 11 100 1.120 60 表 3 0% S不同初始孔隙比下的拟合参数
Table 3. Fitting parameters of 0% S with different initial void ratios
e0 α β 0.633 0.00287 0.719 0.685 0.00256 0.754 0.795 0.00311 0.773 表 4 砂含量不同时无黏性土的拟合参数
Table 4. Fitting parameters of cohesionless soils with different sand contents
试样 k et β 0% S 0.0066 0.258 0.749 20% S 0.0093 0.419 0.776 40% S 0.0105 0.400 0.781 -
[1] 杨光华, 姚丽娜, 姜燕, 等. 基于e–p曲线的软土地基非线性沉降的实用计算方法[J]. 岩土工程学报,2015,37(2):242 − 249. [YANG Guanghua, YAO Lina, JIANG Yan, et al. Practical method for calculating nonlinear settlement of soft ground based on e–p curve[J]. Chinese Journal of Geotechnical Engineering,2015,37(2):242 − 249. (in Chinese with English abstract) doi: 10.11779/CJGE201502005
[2] 曹文贵, 印鹏, 贺敏, 等. 考虑实测数据新旧程度的工后沉降单项模型预测方法[J]. 水文地质工程地质,2015,42(6):65 − 70. [CAO Wengui, YIN Peng, HE Min, et al. A prediction method for post-construction settlement of a single model with the consideration of the new or old degree of the measured data[J]. Hydrogeology & Engineering Geology,2015,42(6):65 − 70. (in Chinese with English abstract)
[3] 曹文贵, 许烜, 李鹏, 等. 基于Eshelby等效夹杂原理的地基沉降分层总和分析方法探讨[J]. 水文地质工程地质,2016,43(6):59 − 65. [CAO Wengui, XU Xuan, LI Peng, et al. A discussion of the layer-wise summation method for ground foundation settlement analysis based on the Eshelby equivalent inclusion[J]. Hydrogeology & Engineering Geology,2016,43(6):59 − 65. (in Chinese with English abstract)
[4] ELKAMHAWY E, ZHOU B, WANG H B. Transitional behavior in well-graded soils: an example of completely decomposed granite[J]. Engineering Geology,2019,253:240 − 250. doi: 10.1016/j.enggeo.2019.02.027
[5] 孙德安, 汪健, 何家浩, 等. 原状扬州黏性土压缩特性与孔径分布[J]. 水文地质工程地质,2020,47(1):111 − 116. [SUN De'an, WANG Jian, HE Jiahao, et al. Compression characteristics and pore-size distributions of the undisturbed Yangzhou clayey soils[J]. Hydrogeology & Engineering Geology,2020,47(1):111 − 116. (in Chinese with English abstract)
[6] 吕亚茹, 李治中, 李浪. 高应力状态下钙质砂的一维压缩特性及试验影响因素分析[J]. 岩石力学与工程学报,2019,38(增刊1):3142 − 3150. [LYU Yaru, LI Zhizhong, LI Lang. One-dimensional compression behavior of calcareous sand and its experimental technology under high stress conditions[J]. Chinese Journal of Rock Mechanics and Engineering,2019,38(Sup1):3142 − 3150. (in Chinese with English abstract)
[7] 王新志, 翁贻令, 王星, 等. 钙质土颗粒咬合作用机制[J]. 岩土力学,2018,39(9):3113 − 3120. [WANG Xinzhi, WENG Yiling, WANG Xing, et al. Interlocking mechanism of calcareous soil[J]. Rock and Soil Mechanics,2018,39(9):3113 − 3120. (in Chinese with English abstract)
[8] MUN W, MCCARTNEY J S. Roles of particle breakage and drainage in the isotropic compression of sand to high pressures[J]. Journal of Geotechnical and Geoenvironmental Engineering,2017,143(10):04017071. doi: 10.1061/(ASCE)GT.1943-5606.0001770
[9] SHENG D C, YAO Y P, CARTER J P. A volume–stress model for sands under isotropic and critical stress states[J]. Canadian Geotechnical Journal,2008,45(11):1639 − 1645. doi: 10.1139/T08-085
[10] MCDOWELL G R, HUMPHREYS A. Yielding of granular materials[J]. Granular Matter,2002,4(1):1 − 8.
[11] BAUER E. Calibration of a comprehensive hypoplastic model for granular materials[J]. Soils and Foundations,1996,36(1):13 − 26. doi: 10.3208/sandf.36.13
[12] 沈珠江. 土的三重屈服面应力应变模式[J]. 固体力学学报,1984,5(2):163 − 174. [SHEN Zhujiang. A stress-strain model for soils with three yield surfaces[J]. Acta Mechanica Solida Sinica,1984,5(2):163 − 174. (in Chinese with English abstract)
[13] 赵颜辉, 朱俊高, 张宗亮, 等. 无黏性土压缩曲线的一种数学模式[J]. 岩土力学,2011,32(10):3033 − 3037. [ZHAO Yanhui, ZHU Jungao, ZHANG Zongliang, et al. A compression model for cohesionless soils[J]. Rock and Soil Mechanics,2011,32(10):3033 − 3037. (in Chinese with English abstract) doi: 10.3969/j.issn.1000-7598.2011.10.023
[14] 王龙, 朱俊高, 郭万里, 等. 无黏性土压缩模型及其验证[J]. 岩土力学,2020,41(1):229 − 234. [WANG Long, ZHU Jungao, GUO Wanli, et al. Compression model for cohesionless soils and its verification[J]. Rock and Soil Mechanics,2020,41(1):229 − 234. (in Chinese with English abstract)
[15] 沈扬, 沈雪, 俞演名, 等. 粒组含量对钙质砂压缩变形特性影响的宏细观研究[J]. 岩土力学,2019,40(10):3733 − 3740. [SHEN Yang, SHEN Xue, YU Yanming, et al. Macro-micro study of compressive deformation properties of calcareous sand with different particle fraction contents[J]. Rock and Soil Mechanics,2019,40(10):3733 − 3740. (in Chinese with English abstract)
[16] 蔡正银, 侯贺营, 张晋勋, 等. 考虑颗粒破碎影响的珊瑚砂临界状态与本构模型研究[J]. 岩土工程学报,2019,41(6):989 − 995. [CAI Zhengyin, HOU Heying, ZHANG Jinxun, et al. Critical state and constitutive model for coral sand considering particle breakage[J]. Chinese Journal of Geotechnical Engineering,2019,41(6):989 − 995. (in Chinese with English abstract)
[17] LEE K L, FARHOOMAND I. Compressibility and crushing of granular soil in anisotropic triaxial compression[J]. Canadian Geotechnical Journal,1967,4(1):68 − 86. doi: 10.1139/t67-012
-



 下载:
下载: